Classical Molecules
xantox, 9 July 2007 in GalleryOther Languages:
Animation showing the interaction of four charges of equal mass1, two positive and two negative, in the approximation of classical electromagnetism. The particles interact via the Coulomb force, mediated by the electric field represented in yellow. A repulsive “Pauli force” of quantum mechanical origin, which becomes very large at a critical distance of about the radius of the spheres shown in the animation, keeps the charges from collapsing into the same point. Additionally, the motion of the particles is damped by a term proportional to their velocity, allowing them to “settle down” into stable (or meta-stable) states.
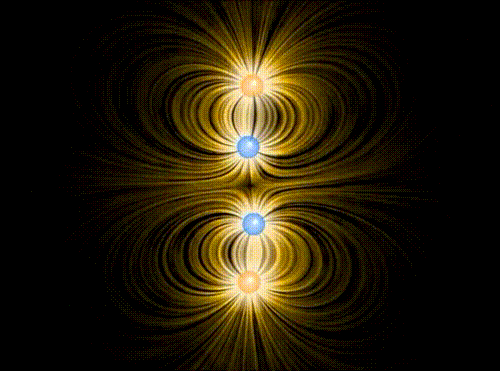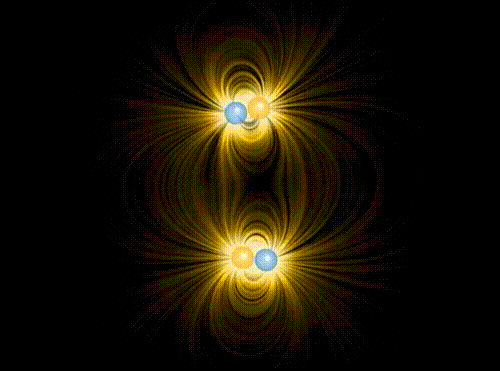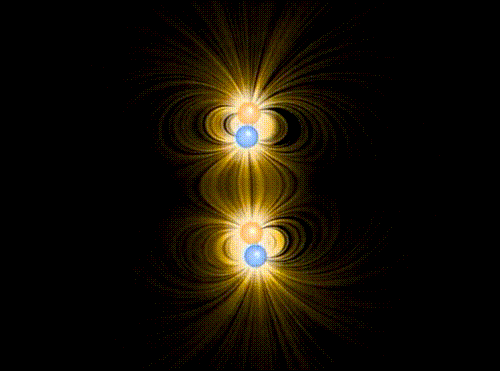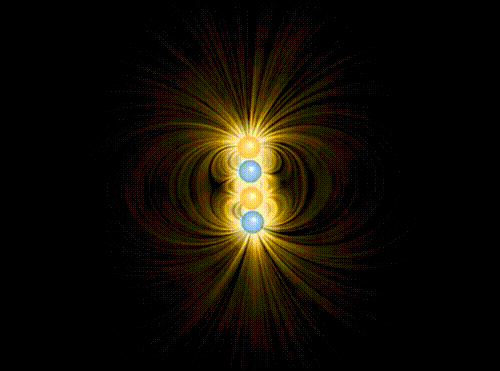
When the charges are allowed to evolve from the initial state, the first thing that happens (very quickly, since the Coulomb attraction between unbalanced charges is very large) is that they pair off into dipoles. Thereafter, there is still a (much weaker) interaction between neighboring dipoles (van der Waals force). Although in principle it can be either repulsive or attractive, there is a torque that rotates the dipoles so that it is attractive, eventually bringing the two dipoles together in a bound state. This mechanism binds the molecules of some substances into a solid.
- © 2004 MIT TEAL/Studio Physics Project, John Belcher [↩]
You can leave a reply, or trackback from your own site.

9 July 2007, 11:39 am
This seems to be a classical simulation, but with a “Pauli force” added in somehow. Can you explain what was done here? Presumably a fully quantum simulation could also be done with the charge distributions, which might be interesting to compare.
10 July 2007, 1:54 pm
The “Pauli” force here is a repulsive force that goes as the inverse of the radial distance r between any two of the charges raised to the power 12. The attractive force between say a positive and negative charge only goes as the inverse of the radial distance between the two charges raised to the power 2. Thus at far distances (far being large compared to the radius of the spheres in the animation) the inverse r squared term dominates and opposite charges will see an attraction and move together, but when they are close enough the inverse r to the 12 term dominates and they are repelled. The inverse r to the 12 term is very “hard”, meaning the transition between attraction and repulsion occurs very rapidly, which is why the charges appear to “bounce” when they collide. The quantum mechanical calculation would show the evolution of the symmetric or anti-symmetric wave functions of the particles, and (1) would be much much much harder to compute and (2) could not be compared directly to the above (I don’t think).
22 September 2007, 11:37 pm
If you did the quantum calculations, you could start with a quantum state of a gaussian wavepacket for each particle, and plot the single particle density function, (integrate the wavefunction squared over all the coordinates except each particle in the system). Of course, this is assuming that the individual particles are distinguishable, which probably wouldn’t be the case in reality. It would still be interesting to watch though.
By the way, nice movie!